What is Finite Element Analysis | Finite Element Modeling? 9 Important Points

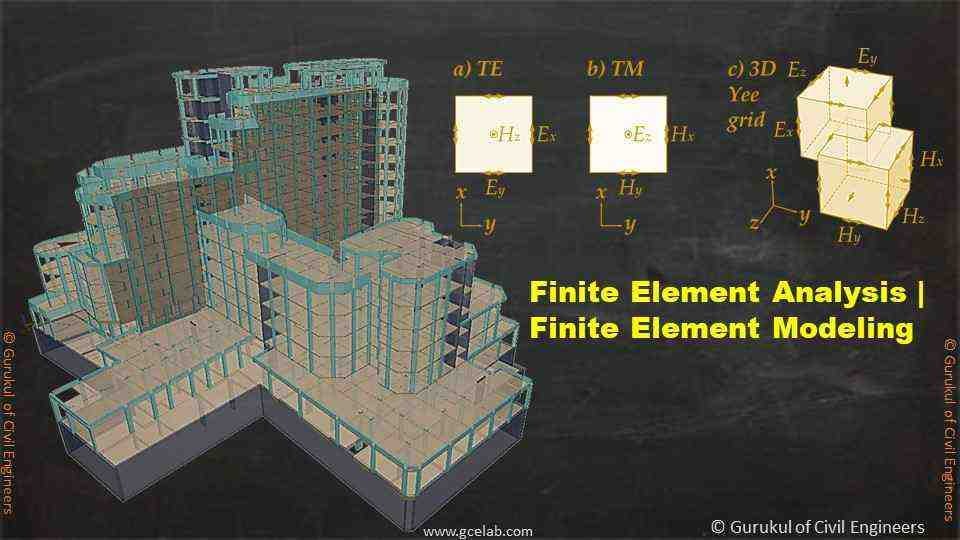
Table of Contents

What is Finite Element Analysis (FEA)?

How does Finite Element Analysis Work?

Why Finite Element Analysis (FEA) is useful?

How to carry out a Finite Element Analysis?

What are the phases/steps of Finite Element Analysis?

Different types of Finite Element Methods?

What are the software’s used for Finite Element Analysis?

Difference between FEA or CFD

Applications of FEA in Civil Engineering

What is Finite Element Analysis (FEA)?

The act of modeling the behaviour of a part or assembly under specified conditions in order to examine it using the finite element method is known as finite element analysis (FEA). Engineers utilize FEA to mimic physical phenomena and eliminate the requirement for actual prototypes while also allowing for component optimization as part of the project design process.

FEA is a method of understanding and quantifying the impacts of real-world conditions on a part or assembly using mathematical models. Engineers can use those mockups, that are run using specialized software, to find possible flaws in a design, such as zones of tension and weak points.

It is likely to comprehend and quantify physical or fluid performance, wave circulation, heat transference, and other processes using mathematics. The majority of procedures can be represented with partial differential equations (PDEs), however, these complicated equations must be fixed in order to determine parameters like strain & stress.

The use of FEA permits for a rough answer to these issues. Recent simulation software is built on the basis of FEA, with the findings being exposed on a computer-generated color scale. While some hypotheses claim that FEA originated with Euler's work in the 16th century, the first mathematical note directly describing the technique came from Schellbach's work in 1851.

Engineers from various businesses around the world continued to develop FEA in order to tackle a wide range of structural procedure problems, particularly in aerospace and civil engineering. FEA for real-world applications was originally created in the mid-fifties, and it was progressively developed throughout the next few decades.

How does Finite Element Analysis Work?

FEA simulations are made up of a mesh of loads of tiny pieces that combine to form the geometry of the assembly under consideration. Each of these minor pieces is calculated separately, with the mesh refinements compounding to generate the overall structure's ultimate output.

These approximation calculations are typically polynomial in nature, with interpolations occurring transversely the small elements, allowing values to be established at some places. The sites where the values may be resolute are known as nodal points, and they are frequently situated near the element's boundary.

Why Finite Element Analysis (FEA) is useful?

Engineers can use FEA to simulate a design in action without having to build a physical model. There are a few more advantages to FEA because of its nature. Finite element modeling provides for the simulation of many material types, the testing of complicated geometry, and the recording of local effects acting on a limited area of the design.

Engineers can utilize finite element modeling software for a wide range of jobs in practice. A car's deformation on impact, human joint strains, and fluid dynamics over turbines are just a few of the many uses. Finite element analysis is merely a model for forecasting. It does not guarantee that a design will withstand the simulated stresses.

However, it provides engineers with an improved understanding of how the design will respond to stressors and lowers the need for extensive prototyping.

How to carry out a Finite Element Analysis?

Finite element analysis (FEA) is a computerized method for predicting how a product will react to forces, vibrations, heat, fluid movement, and other physical influences in the actual world. Finite element analysis determines if a product will, wear out, break or function as intended.

Read More:

• What is the Function of Bridge Foundation or Pier and its Types? 5 Important Points
• 10 Latest Type of Bridges and its components

What are the phases/steps of Finite Element Analysis?

The finite element method is a mathematical procedure for getting solutions to boundary-value problems, as described in the introduction. The approach works by replacing a whole continuous domain with a series of subdomains, each of which represents the unknown function with simple interpolation functions with unknown coefficients.

As a result, the original boundary-value problem with an infinite number of degrees of freedom is reduced to a problem with a finite number of degrees of freedom, or in other words, the full system's solution is approximated by a finite number of unknown coefficients. As a result, the following should be included in a finite element analysis of a boundary-value problem:

• “Discretization or subdivision of the domain is a term used to describe the process of dividing a domain.
• The interpolation functions are chosen (to provide an estimate of the unknown resolution within an element.
• The major stage in FEM is the design of the system of calculations. It's possible to apply the standard Ritz variational & Galerkin approaches.)
• The system of equations is resolved. (Once the system of equations has been solved, the anticipated parameters can be computed and displayed in the form of curves, graphs, or color graphics, that are more intelligible and understandable”.

Different types of Finite Element Methods?

FEA may be used to gain structural and mechanical validation in a variety of sectors. In some circumstances, mechanical certification is contingent on the completion of an FEA. The use of Finite Element Analysis is crucial in the following situations:

• Load capacity certification for hoisting cranes
• Machine and machine part fatigue analysis
• Platform assistance is available.
• Lifetime certification for brakes or rotors
• Validation and forensic analysis
• Analysis of a pressure vessel
• Bridges across airports
• Design of the machine”

What are the software’s used for Finite Element Analysis?

Following are the top software packages used in FEA:

• COMSOL Multiphysics. COMSOL Multiphysics finite element analysis software is renowned for its connectivity with other software such as MATLAB, Java, and Autodesk Inventor
• Open Foam
• ABAQUS
• Altair Hyper Works
• Autodesk CFD
• LS-DYNA”

Difference between FEA or CFD

The distinction between FEA and CFD is nuanced. Finite Element Analysis (FEA) is a method of solving partial differential equations that have traditionally been used to solve structural issues. Computational Fluid Dynamics (CFD) is a collection of approaches that are similar but better suitable for handling fluid-flow issues.

Applications of FEA in Civil Engineering

• In the civil engineering discipline, finite element analysis (FEA) is a very important tool for mathematically approximating structures that are complicated for standard analytical solutions. Consider a concrete beam with support from both ends that is subjected to an intense load in the middle span. Because the beginning and edge conditions are finite and under control, the deflection at the centre span may be calculated numerically in a reasonably straightforward manner. When the same beam is transported into a real-time application, e.g.- bridge, the forces become very difficult to analyze using simple mathematics.
• There are various problems in structural engineering where FEA could be applied to simplify a structure and comprehend its complete behaviour.
• As the discipline of computer-aided engineering (CAE) has progressed, so has the turf of finite element analysis (FEA), which has benefited the civil engineering industry to a very great extent. Cutting-edge FEA tools have not only resulted in more inventive and efficient outputs but have also aided in the development of precise design procedures.

I hope the above blog provides you with an in-depth knowledge of Finite Element Analysis | Finite Element Modeling.

Please feel free to like, share and comment.  

Read More:

• What is Honeycombing of Concrete? Prevention and Repair: 8 Important Points
• Pre-stressed Concrete, Pre-tensioning and Post-tensioning: 3 Important Points
• What is Earthquake Resistant Building | Structure? 8 Important Points

Admin, gcelab.com
Please see our Pillar Post to know why we founded gcelab.com.

Pooja

Founder at gcelab.com, Pooja is an Entrepreneur unlocking human potential. Working in the Principles of Lean Start-up, Pooja believes in Transparency and User Happiness the most. Pooja’s background in teaching gives her a sophisticated grasp on even the most tedious aspect of course building. She is passionate about people who believe that good is not enough.